Senin, 16 Desember 2019
Minggu, 15 Desember 2019
Simak Ui 2009 (Semua Kode)
nah kali ini Math-lab hendak berbagi soal SIMAK UI 2009 Super lengkap, semua kode soal dalam satu file. semoga bermanfaat beringsang beringsang
Download disini
Simak Ui 2014 (Matematika Dasar)
kepada kesempatan kali ini Math-lab hendak berbagi soal Matematika Dasar SIMAK UI 2014. Semoga Bermanfaat.
Download disini
Buku Kurikulum 2013 Kelas 12 (Matematika Wajib)
dengan pelajaran matematika Kurikulum 2013 banyak materi baru yg ditambahkan yg sebelumnya tidak ada dengan kurikulum KTSP, salah satunya adalah materi tentang Matematika Keuangan kelas 12. bagi yg ingin mempelajarinya, silahkan download buku berikut ini:
atau Download disini
Rpp Kurikulum 2013 Matematika Wajib Kelas X
Kali ini Math-lab bakal berbagi Rencana Pelaksanaan Pembelajaran (RPP) Kurikulum 2013 untuk Pelajaran Matematika wajib Kelas X. beringsang
silahkan download dengan link berikut:
1. RPP Eksponen lagi Logaritma
2. RPP Persamaan lagi Pertidaksamaan Nilai Mutlak
3. RPP Persamaan lagi Pertidaksamaan Linear
4. RPP Sistem Persamaan Linear
5. RPP Relasi lagi Fungsi
6. RPP Matriks
untuk sementara hanya bisa berbagi segitu dulu, insyaAllah lain kesempatan bakal di update lagi.
semoga bermanfaat
Sabtu, 14 Desember 2019
Ringkasan Materi Persamaan Kuadrat
Langsung aja deh, inilah ringkasan materi Persaan Kuadrat
bergolak
atau Download disini.
setelah anda mempelajari materinya, silahkan perbanyak latihan soal ataupun bisa juga mencoba soal persamaan kuadrat yg sudah Mathlab siapkan pada link ini
Download Soal Persamaan Kuadrat
Sebelumnya Mathlab ucapkan selamat datang di blog sederhana ini, dengan kesempatan kali ini Mathlab hendak berbagi soal tentang Persamaan Kuadrat, bila Mathlab sarankan sebelum mencoba soal-soal ini alangkah baiknya kalian pelajari dulu ringkasan materinya yg sudah Mathlab sediakan di link berikut ini . dan ini soalnya:
Download Soal Dimensi Tiga
Mohon maaf postingan soal dimensi tiga (bangun ruang) ini sudah saya hapus.
silakan untuk mengunduh soal dimensi tiga terbaru kepada link di bawah ini:
Download Soal Dimensi Tiga (Bangun Ruang) Matematika Wajib Kelas 12
Semoga bermanfaat.
silakan untuk mengunduh soal dimensi tiga terbaru kepada link di bawah ini:
Download Soal Dimensi Tiga (Bangun Ruang) Matematika Wajib Kelas 12
Semoga bermanfaat.
Download Soal Fungsi Kuadrat
gerah
sebelum mengerjakan soal Fungsi Kuadrat yg bagi Mathlab berikan, sebaiknya kalian pelajari dulu ringkasan materinya pada link berikut ini. Jika kalian sudah mempelajarinya, langsung aja inilah soal-soal Fungsi Kuadrat:
Jumat, 13 Desember 2019
Download Diktat Olimpiade Matematika Demam Sma
kepada kesempatan kali ini Mathlab akan berbagi diktat olimpiade matematika SMA karya Bapak Eddy Hermanto, ST, semoga diktat ini bermanfaat buat adik-adik yg sedang mempersiapkan diri buat mengikuti olimpiade bahang bahang
download disini
Menentukan Jumlah Deret Aritmetika Bertingkat
Misalkan ada barisan $u_1, u_2, u_3, \cdots, u_n$ merupakan barisan dengan selisih tidak konstan. tetapi apabila diambil $D_1(n)=S_n-S_{(n-1)}$ lalu $D_2(n)=D_1(n)-D_1(n-1)$ bersama seterusnya sampai kepada suatu saat $D_k(n)=D_{k-1}(n)-D_{k-1}(n-1)$ bernilai konstan. Maka bisa kita ambil kesimpulan bahwa rumus jumlah $n$ suku pertama $S_n$barisan tersebut merupakan polinomial pangkat $k$.
Contoh:
Diketahui barisan 3, 6, 10, 15, 21, ... tentukan jumlah n suku pertama $S_n$ !
Solusi:
Jika kita perhatikan barisan bilangan 3, 6, 10, 15, 21, ... bukanlah merupakan barisan aritmetika karena selisihnya tidak konstan. Namun misalnya kita perhatikan selisihnya sebagai berikut:
Dari kedua tabel didapat bahwa:

dari persamaan (2) diperoleh





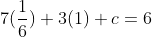





dari persamaan ke (4)






maka persamaan jumlah deret tersebut adalah


Jika Anda sudah memahami langkah-langkah menentukan jumlah deret aritmatika bertingkat seperti yg dijelaskan di atas, anda boleh mencoba menyelesaikan soal berikut:
Contoh:
Diketahui barisan 3, 6, 10, 15, 21, ... tentukan jumlah n suku pertama $S_n$ !
Solusi:
Jika kita perhatikan barisan bilangan 3, 6, 10, 15, 21, ... bukanlah merupakan barisan aritmetika karena selisihnya tidak konstan. Namun misalnya kita perhatikan selisihnya sebagai berikut:
atau bisa kita tulis dalam tabel sebagai berikut:
ternyata kepada tingkatan ketiga ataupun kepada tabel $D_3(n)$ selisihnya konstan. maka bisa disimpulkan bahwa jumlah suku ke $n$ ataupun $S_n$ barisan tersebut merupakan polinomial berderajat 3.
misalkan :
$S_n=an^3+bn^2+cn+d$
maka kita peroleh:
Dari kedua tabel didapat bahwa:
dari persamaan (1) diperoleh :
dari persamaan (2) diperoleh
dari persamaan (3) diperoleh
dari persamaan ke (4)
maka persamaan jumlah deret tersebut adalah
Jika Anda sudah memahami langkah-langkah menentukan jumlah deret aritmatika bertingkat seperti yg dijelaskan di atas, anda boleh mencoba menyelesaikan soal berikut:
Pembahasannya download disini
Baca juga: Konsep barisan aritmetika bertingkat
Ketaksamaan Rataan Kuadrat (Qm), Rataan Aritmatika (Am), Rataan Geometri (Gm) Kepada Rataan Harmonik (Hm)
Mungkin kita agak asing dengan istilah-istilah Ketaksamaan Rataan Kuadrat (QM), Rataan Aritmatika / Arithmatic Mean (AM), Rataan Geometri / Geometric Mean (GM) beserta Rataan Harmonik / Harmonic Mean (HM), baiklah kalau gitu bakal saya jelaskan dulu satu persatu
Secara umum, Jika kita mempunyai n buah bilngan maka di definisikan bahwa:
maka di definisikan bahwa:
Secara umum, Jika kita mempunyai n buah bilngan
beserta selalu berlaku :
Contoh Soal 1:
(Soal Kompetensi Matematika SMU Bandung 1998/1999)
untuk p, q, r > 0 dan p+q+r=1 , buktikan bahwa
Jawab:
Terbukti
Contoh Soal 2:
Buktikan bahwa  untuk bilangan real positif a dan b.
untuk bilangan real positif a dan b.
Jawab:
berdasarkan ketaksamaan AM-GM diperoleh:
Mathsmagic: Multiplication Showing Double Digit Dexterity
Most people can do a speedy multiply by 10. You just add a zero to the end of the number – 23 multiplied by 10 is 230, simple. Now you can prove your superior mental superpowers by speedy multiplication of any two-digit number by 11. You explain to the audience that this is clearly far more difficult. They have the calculators on their mobile phones ready to check, but you do your calculations correctly before they even start to click the keys.
Imagining the 11 times trick
To give us this superpower, we make use of two things. One is maths and the other is the human brain’s power of imagination.
To do a lightning calculation multiplying any two-digit number by 11, you need to use some visual imagery and use your imagination. Let’s take the number 52 for example. Now imagine a space between the two digits, so in your minds eye you imagine 5 2. Add the two numbers together and imagine putting the sum of them in the gap in the middle, so you see 5(5+2)2. And that is it, you have the answer: 11x 2 = 5(7)2= 572.
The double trouble trick
But what if the numbers in the gap add up to a double digit? For example, suppose you want to multiply 98 by 11. So, you imagine 9 (9+8) 8. But that bit in gap in the middle gives you 9+8=17, so where do you put these digits? 9 (17) 8?
Easy, just leave the second number (here the 7) in the gap as before and imagine moving the 1 up a place, so you have (9+1) 7 9 = 10 7 9 = 1079. Correct again.
The maths behind this is fairly easy if you explore it. Suppose you have the number AB (that’s A tens and B ones) and you want to multiply by 11. First you multiply by 10. That’s easy, 10xAB = AB0 (A hundreds, B tens and 0 ones). Then you add another AB so you’ve got 11 lots of AB altogether, giving you A hundreds, (B+A) tens and (0+B) ones.
This is exactly what all that sliding numbers around in your imagination has been doing without knowing it. Of course, if the middle A+B is more than 10 (ie it’s a double digit number), you just slide the first digit up to the hundreds column, and it’s sorted.
Lightning calculations by mind power and a maths trick. Mathematicians make use of their imaginations all the time. Our brains are really good at imagining things and creating pictures in our heads. Often that’s the way we solve tricky problems or come up with clever visual imagination tricks like this one.
panas Kamis, 12 Desember 2019
Mathsmagic : Multiplication And Addition Doing Fibonacci’S Lightning Calculation
On a piece of paper, write the number 1 to 10 in a column. You are now all set to amaze with the speed at which you can add ten numbers.
Ask your friend to choose any two two-digit numbers and write the numbers down in the first two spaces of your column, one under the other. Your friend then makes a third number by adding these first two numbers together and writes it below the first two, in effect starting a chain of numbers. They make a fourth number by adding the second and third, a fifth by adding the third and fourth, and so on, until your column of ten numbers is full.
To show how brilliant you are, you can turn away once your friend has understood the idea, say after the seventh number in the list. Now you can’t even see the numbers being written.
Meanwhile, with your back turned, you are actually multiplying that seventh number by 11 to get the final answer.
Let’s imagine your friend chose 16 and 21 to start with. The list would look like this:

You now turn round and write the sum of all ten numbers straight away! Lightning quick, you say it is 2728. Let them do it slowly on a calculator to show your brilliant mind skills are 100 per cent correct. The final answer just involves multiplying the seventh number by 11. Why?
Well this chain of numbers where the next term is made by adding the previous two terms is called a Fibonacci sequence. Fibonacci sequences have special mathematical properties that most folk don’t know about…
So let’s look at the trick. We start with the two numbers A and B. The next number is A+B, the next number is B added to A+B which is A+2B and so on. Going through the number chain we find:
Adding up all 10 numbers in the chain gives us a grand total of 55A+88B – check it yourself. But look at the seventh number in your column… this line is 5A+8B. It is exactly the total of the chain but divided by 11!
So working backwards, you can get the final total by multiplying the seventh term by 11. And the maths proves this lightning calculation will work for any two starting values A and B.
It is up to you to present this trick in such a way that it looks like you are just very, very clever. Which of course you are, as you now know how to use a Fibonacci sequence for magic.
bergolak
Langganan:
Postingan (Atom)


















